Problem of The Day: Valid Sudoku

Problem Statement

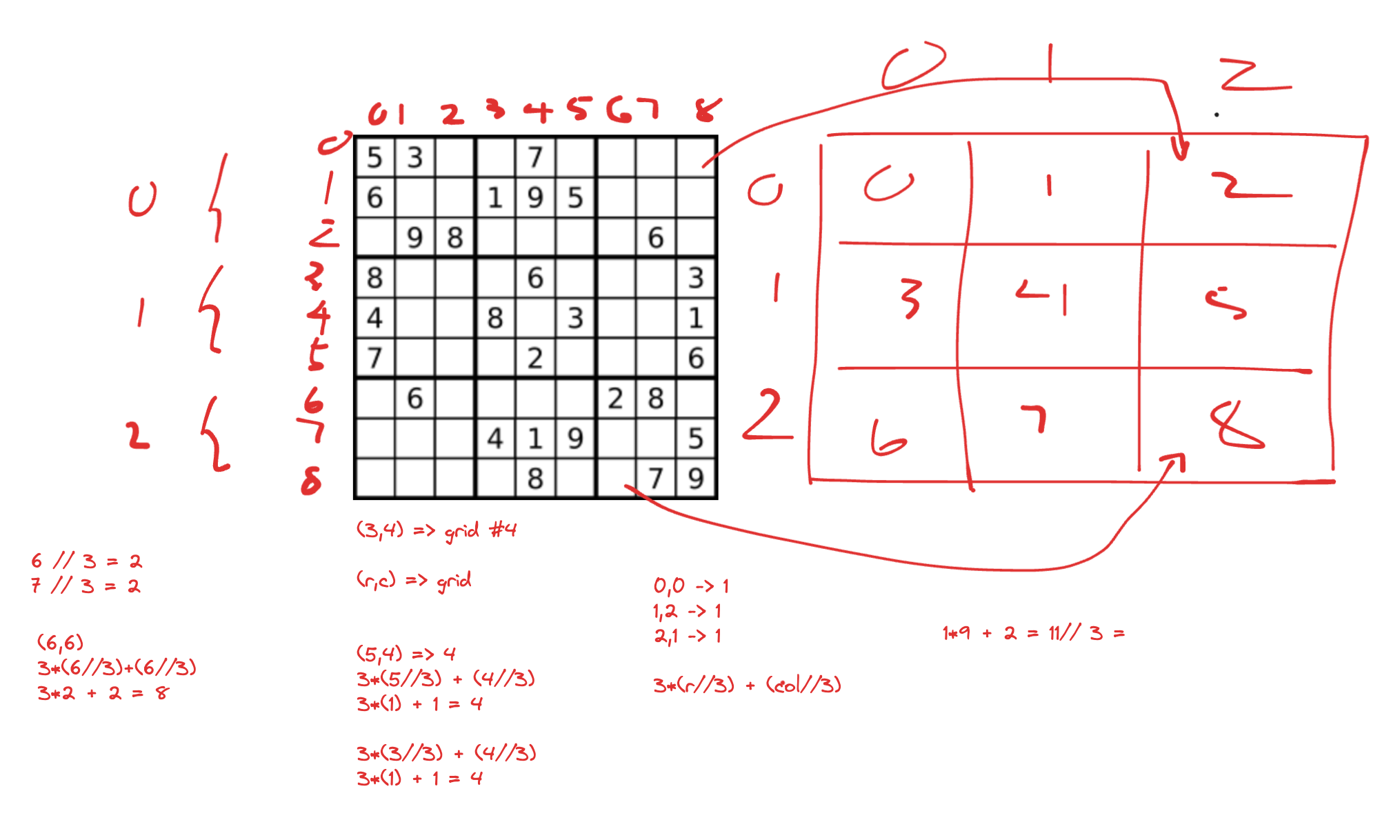
My note:

Intuition

The problem requires checking whether a given 9x9 Sudoku board is valid. A valid Sudoku board should satisfy the rules that each row, each column, and each of the 9 sub-grids that compose the board should contain all of the digits from 1 to 9 without repetition.

Approach

I’ve defined three helper functions (check_rows, check_cols, and check_grids) to check the validity of rows, columns, and sub-grids, respectively. Each function iterates through the corresponding elements and uses a set to keep track of seen digits. If a digit is encountered more than once in a row, column, or sub-grid, the board is considered invalid.

The main function isValidSudoku then calls these three helper functions and returns the logical AND of their results. If all three conditions are met (rows, columns, and sub-grids are valid), the Sudoku board is considered valid.

Complexity

• Time complexity: O(n^2), where n is the size of the Sudoku board (9x9). The function iterates through each cell in the board to check rows, columns, and sub-grids.

• Space complexity: O(n), as additional space is used to store sets for checking each row, column, and sub-grid.

Code

class Solution:
    def isValidSudoku(self, board: List[List[str]]) -> bool:
        ROWS = COLS = 9

        def check_rows(mat):
            for r in range(ROWS):
                seen = set()
                for c in range(COLS):
                    if mat[r][c] != "." and mat[r][c] in seen:
                        return False
                    seen.add(mat[r][c])
            
            return True
        

        def check_cols(mat):
            for c in range(COLS):
                seen = set()
                for r in range(ROWS):
                    if mat[r][c] != "." and mat[r][c] in seen:
                        return False
                    seen.add(mat[r][c])

            return True

        
        def check_grids(mat):
            grid = defaultdict(set)
            for r in range(ROWS):
                for c in range(COLS):
                    idx = 3*(r//3) + (c//3)
                    if mat[r][c] != "." and mat[r][c] in grid[idx]:
                        return False
                    grid[idx].add(mat[r][c])
            return True

        return check_rows(board) and check_cols(board) and check_grids(board)

Editorial Solution

clean code

class Solution:
    def isValidSudoku(self, board: List[List[str]]) -> bool:
        N = 9

        # Use hash set to record the status
        rows = [set() for _ in range(N)]
        cols = [set() for _ in range(N)]
        boxes = [set() for _ in range(N)]

        for r in range(N):
            for c in range(N):
                val = board[r][c]
                # Check if the position is filled with number
                if val == ".":
                    continue

                # Check the row
                if val in rows[r]:
                    return False
                rows[r].add(val)

                # Check the column
                if val in cols[c]:
                    return False
                cols[c].add(val)

                # Check the box
                idx = (r // 3) * 3 + c // 3
                if val in boxes[idx]:
                    return False
                boxes[idx].add(val)

        return True